Calculation of the velocities of two consecutive parabolic movements and calculation of the intensity of the generated sound (ESP-ENG)

When we attend any sports field we can notice the development of multiple movements, but above all, of those made by the object with which a certain sport is carried out, for example, a soccer ball, basketball, American soccer, goal ball, among many other objects, such as the throwing of a javelin, a disc, an arrow, and in this opportunity we will calculate the velocities of two consecutive parabolic movements, which manage to develop in the sports discipline called baseball, In this opportunity we will calculate the velocities of two consecutive parabolic movements, which are developed in the sport discipline called baseball, and we will also calculate the intensity of the sound intensity originated by the impact of the ball with the bat.
How many times have we seen the throwing of a curve from a pitcher to a batter who hits the ball with his bat, both movements describe the trajectory of a parabola and do it consecutively one after the other, and of course, both movements developed with different speeds, and that is what we propose to do in this article by implementing the interpretation of physical science through the algebraic formulations offered by the essential field of mathematics.
Therefore, it is important that we continue describing practical exercises in the sports área in order to verify that science is an essential part of these disciplines that transmit so many emotions and, in addition, help us to maintain an adequate physical-mental health, then, next, I show you the statement related to the calculation of the two speeds described above in relation to two consecutive parabolic movements.
Exercise
In a baseball stadium a large number of spectators or fans of this sport, are enjoying an exciting game, when they observe that the pitcher perform a curveball thanks to the grip of the ball and the rotating movement of his wrist, the angle at the time of releasing the ball was 30°, and the distance between the pitcher and the receiver is 60 feet and 6 inches (18.44 m), whose arrival time of the curveball at home plate is 0.75 seconds, 44 m), whose time of arrival of the pitch in curve to the home plate is 0.75 seconds, however, it does not manage to surprise the batter who connects the ball, the ball when impacting with the bat leaves with an angle of 45°, and takes a great speed with which it manages to leave the park in a time of 2.5 seconds, the truth is that the ball developed two consecutive curvilinear or parabolic movements.
All spectators enjoy the sound originated by the impact of the ball with the bat, a group of experts in acoustics determined that the acoustic power (P) of such impact (ball-bat) was 0.003 W, in relation to the above described, answer the following questions:
a.- What was the speed of the pitching of the ball by the pacer?
b.- What was the velocity of the ball hit with the bat knowing that the ball traveled 384 feet (117.04 m)?
c.- What was the sound intensity generated by the impact of the ball with the bat if the person who calculated its power (P) was 8 m away from the batter?
Solution
Data:
θ1 = 30° (Pitching angle of the ball by the pigeon).
θ2 = 45° (Angle of ball launch by the bat).
dH1 = 18,44 m (Horizontal distance between pacer and batter).
dH2 = 117,04 m (Horizontal distance traveled by the batted ball).
t1 = 0,75 s (Time to reach home plate).
t2 = 2,5 s (Time taken for the ball to be hit by the bat and leave the park).
P = 0,003 W. (Power generated by the sound of the bat hitting the ball).
r = 8 m (Distance between the sound source and the sound receiver).
V1 = ? (Speed of the ball thrown by the pacer).
V2 = ? (Speed of the ball when hit by the bat).
I = ? (Sound intensity).
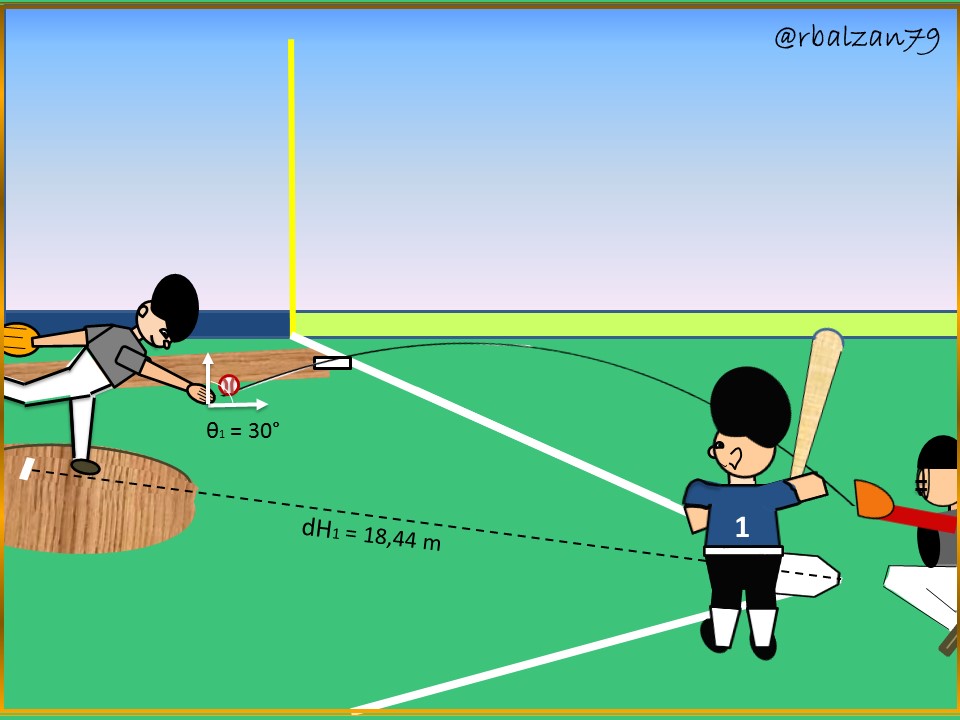
a.- We begin to analyze our first unknown, which is related to the speed of the pitcher's throw of the ball and, for this, we could implement the formula for the horizontal distance and then look for the requested variable, therefore, we proceed as follows:

This represents the speed of the pitcher's throw in m/s, km/h and mph, characteristic speed of a curvilinear throw and which describes the trajectory of a parabola as observed in the gif of the article presentation.
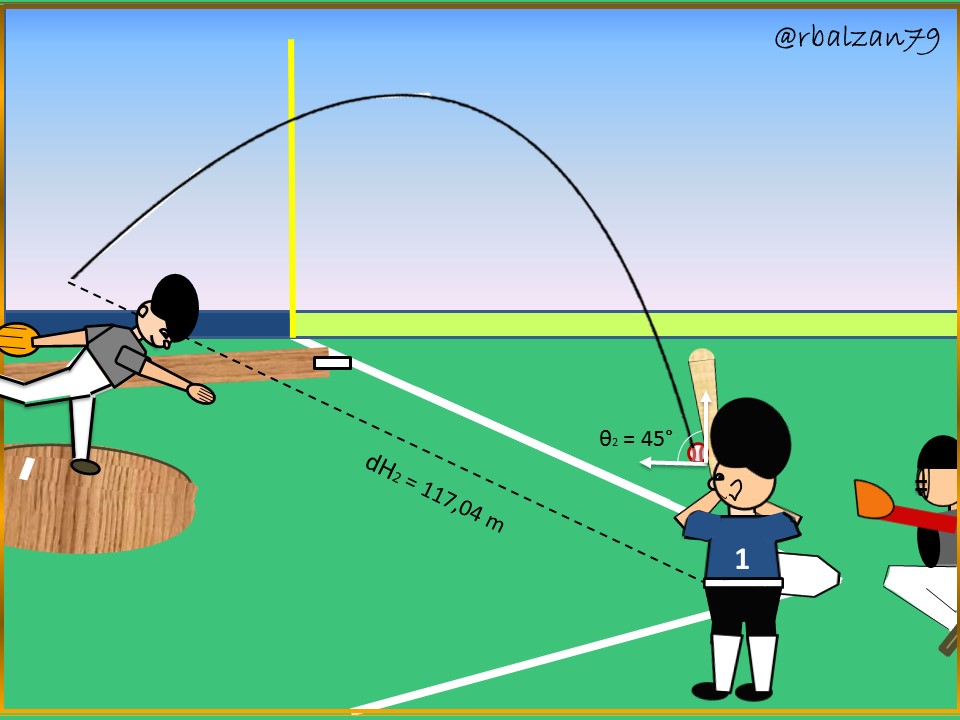
b.- Now we can proceed in the same way to find the velocity with which the ball travels when hit by the bat, describing in the same way a parabola, so we can proceed as follows:
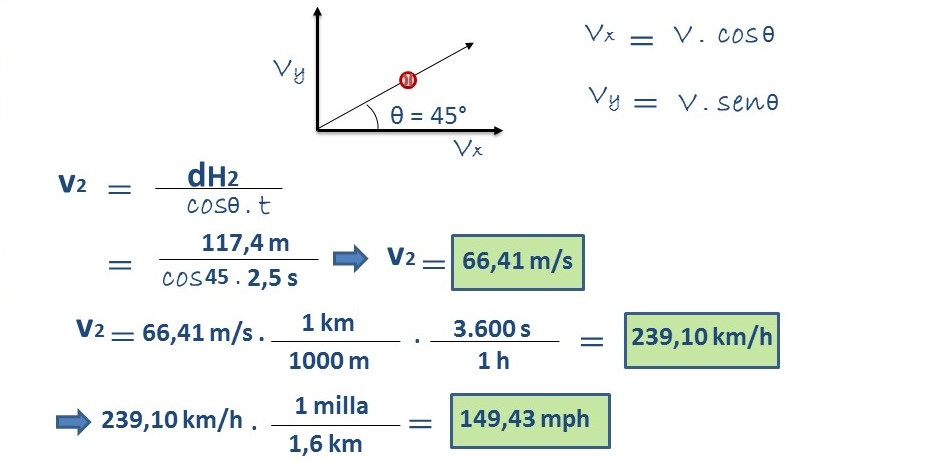
The previous value represents the speed with which the ball was shot upon impact with the bat, logically it is greater than the speed achieved by the pitcher, since it is the sum of the force contributed by the batter, plus the speed with which the ball comes by the pitcher, even though it is a curveball, therefore, slow, the power of the batter manages to inject enough speed for the ball to leave the stadium.
c.- Now we have to calculate the intensity of the sound generated by the impact of the ball with the bat, so we proceed using the data we have and we will do this by implementing the following formulation:

In this way we are able to know the intensity of the sound generated by the impact of the bat with the ball thrown by the pitcher, both actions describing successive parabolic movements.
Conclusion
Undoubtedly one of the most known movements by man is the curvilinear, in its parabolic version as those analyzed above, and they are developed consecutively in a baseball game, and both are fascinating for the spectators, of these parabolic movements we can calculate their speeds, one greater than the other because the bat gives the ball the force of the batter, and also takes into account the force with which the pitcher throws the ball, even though the pitch is a curve.
There are many changes of rhythm in relation to the speed of the ball that a pitcher makes, and apart from the straight throw, all the others describe a parabola from lesser to greater intensity depending on the type of throw, but, above all, the curve is the best expression of a beautiful parabolic trajectory, there are many examples that we could find of curvilinear movements, especially parabolic, and they are an essential part of our lives.
Until another opportunity my dear friends.
Note: The images were created by the author @rbalzan79 using Power Point and Paint, the animated image was created using the PhotoScape application.
Recommended bibliographic references
[1] Parabolic Projectiles. Link.
[2] El movimiento rectilíneo. Link.
[3] Projectile Motion. Link.
Spanish version
Cuando asistimos a cualquier campo deportivo podemos notar el desarrollo de movimientos múltiples, pero sobre todo, de aquellos realizado por el objeto con el cual se lleva determinado deporte, por ejemplo, un balón de futbol, basquetbol, futbol americano, pelota de gol, entre tantos otros objetos, como el lanzamiento de una jabalina, de un disco, una flecha, y, en esta oportunidad calcularemos las velocidades de dos movimientos parabólicos consecutivos, los cuales, logran desarrollarse en la disciplina deportiva denominada el beisbol y además, calcularemos la intensidad del sonido originado por el impacto de la pelota con el bate.
Cuantas veces hemos visto el lanzamiento de una curva de un lanzador hacia un bateador el cual golpea dicha pelota con su bate, ambos movimientos describen la trayectoria de una parábola y lo hacen consecutivamente una después del otro, y por supuesto, ambos movimientos desarrollados con distintas velocidades, y eso, es lo que nos proponemos a realizar en este artículo al implementar la interpretación de la ciencia física a través de las formulaciones algebraicas ofrecidas por el imprescindible campo de las matemáticas.
Por lo tanto, es importante que sigamos describiendo ejercicios prácticos en el área deportiva con la finalidad de verificar que la ciencia es parte esencial de estas disciplinas que tantas emociones nos transmiten y, que, además, nos ayudan a mantener una adecuada salud física-mental, entonces, a continuación, les muestro el enunciado relacionado al cálculo de las dos velocidades antes descritas en relación a dos movimientos parabólicos consecutivos.
Ejercicio
En un estadio de beisbol una gran cantidad de espectadores o fanáticos a este deporte, se encuentran disfrutando de un emocionante juego, cuando observan que el pícher realizar un lanzamiento en curva gracias al agarre de la pelota y el movimiento giratoria de su muñeca, el ángulo al momento de soltar la pelota fue de 30°, y, la distancia entre el pícher y el receptor es de 60 pies y 6 pulgadas (18,44 m), cuyo tiempo de llegada del lanzamiento en curva al home plate es de 0,75 segundos, sin embargo, no logra sorprender al bateador el cual conecta la bola, la pelota al impactar con el bate sale con un ángulo de 45°, y toma una gran velocidad con la cual consigue salir del parque en un tiempo de 2,5 segundos, lo cierto es que la bola desarrollo dos movimientos curvilíneos o parabólicos consecutivos.
Todos los espectadores disfrutan del sonido originado al impactar la bola con el bate, un grupo de expertos en acústica logra determinar que la potencia acústica (P) de dicho impacto (bola-bate) fue de 0,003 W, en relación a lo antes descrito, responder a las siguientes interrogantes:
a.- ¿Cuál fue la velocidad del lanzamiento de la bola por el pícher?
b.- ¿Cuál fue la velocidad de la bola impactada con el bate sabiendo que la misma recorrió 384 pies (117,04 m)?
c.- ¿Cuál fue la intensidad sonora generada por el impacto de la bola con el bate si la persona que calculo su potencia (P) se encontraba a 8 m del bateador?
Solución
Datos:
θ1 = 30° (Ángulo de lanzamiento de la bola por el pícher).
θ2 = 45° (Ángulo de lanzamiento de la bola por el bate).
dH1 = 18,44 m (Distancia horizontal entre pícher y bateador).
dH2 = 117,04 m (Distancia horizontal recorrida por la bola bateada).
t1 = 0,75 s (Tiempo en llegar la bola al home plate).
t2 = 2,5 s (Tiempo implementado por la bola al ser impactada por el bate y salir del parque).
P = 0,003 W. (Potencia generada por el sonido del impacto del bate con la bola).
r = 8 m (Distancia entre el foco emisor del sonido y receptor del mismo).
V1 = ? (Velocidad de la bola lanzada por el pícher).
V2 = ? (Velocidad de la bola al ser golpeada por el bate).
I = ? (Intensidad del sonido).

a.- Comenzamos a analizar nuestra primera incógnita la cual esta relacionada con la velocidad del lanzamiento de la pelota por el pícher y, para ello, podríamos implementar la fórmula de la distancia horizontal para luego buscar la variable solicitada, por lo tanto, procedemos de la siguiente manera:

Esta representa la velocidad del lanzamiento del pícher tanto, en m/s, km/h y mph, velocidad característica de un lanzamiento curvilíneo y el cual describe la trayectoria de una parábola como se ha observado en el gif de la presentación del artículo.

b.- Ahora podemos proceder de la misma manera a encontrar la velocidad con la cual la pelota viaja al ser golpeada por el bate, describiendo de igual forma una parábola, así que podemos proceder de la siguiente manera:

El anterior valor representa la velocidad con la que salió la bola disparada al impactar con el bate, lógicamente es mayor que la velocidad lograda por el pícher, ya que es la suma de la fuerza aportada por el bateador, más la velocidad con la que viene la bola por el pícher, con todo que es un lanzamiento en curva, por lo tanto, lento, el poder del bateador logra inyectarle la velocidad suficiente para que la bola salga del estadio.
c.- Ahora nos queda calcular la intensidad del sonido generado por el impacto de la bola con el bate, así que procedemos utilizando los datos con cuales contamos y esto lo haremos implementando la siguiente formulación:

De esta manera logramos conocer la intensidad del sonido generado por el impacto del bate con la bola lanzada por el pícher, ambas acciones describiendo movimientos parabólicos sucesivos.
Conclusión
Sin duda alguna uno de los movimientos más conocidos por el hombre es el curvilíneo, en su versión parabólico como los que analizamos anteriormente, y los mismos se desarrollan consecutivamente en un juego de beisbol, y ambos son fascinantes para los espectadores, de estos movimientos parabólicos logramos calcular su velocidades, una mayor que la otra debido a que el bate le imprime a la pelota la fuerza del bateador, y también toma en cuenta la fuerza con la que el pícher lanza la bola, a pesar que dicho lanzamiento sea una curva.
Muchos son los cambios de ritmos en relación a la velocidad de la pelota que un pícher realiza, y apartando el lanzamiento en recta, todos los demás describen una parábola de menor a mayor intensidad dependiendo del tipo de lanzamiento, pero, sobre todo la curva es la mejor expresión de una hermosa trayectoria parabólica, muchos son los ejemplos que podríamos encontrarnos de movimientos curvilíneos, en especial parabólicos, y los mismos formando parte esencial de nuestras vidas.
Hasta otra oportunidad mis queridos amigos.
Nota: Las imágenes fueron creadas por el autor @rbalzan79 usando Power Point y Paint, la imagen animada fue creada usando la aplicación PhotoScape.
Referencias bibliográficas recomendadas
[1] TIRO PARABOLICO. Link.
[2] Projectile Motion. Link.
This topic should be on projectile motion if I am not mistaken
Hello thank you for commenting, the parabolic motion is not only limited to the analysis of projectile shooting, next to us occur countless phenomena that develop a parabolic path, as described in this article, and so in other sports disciplines, including activities such as projectile shooting.