Another Interesting Conclusion from a Non-hypotenuse Side and In-radius of a Right Triangle
Hello math bugs(🐞) and hivers(🐝)
I hope everything with okay with you.
Well come to another geometric problem and it's solution.
We have a right angle triangle. If in-radius(r) of circle inside the triangle and a side (a=non hypotenuse) are given, we can find the area using both of them. Area in terms of the a and r can be given by : ∆= ar(a-r)/(a-2r)

To prove the above consideration, what we need are follows:
☑️ Lentgh of a common tangents from a fixed point outside a circle are equal in lenght:
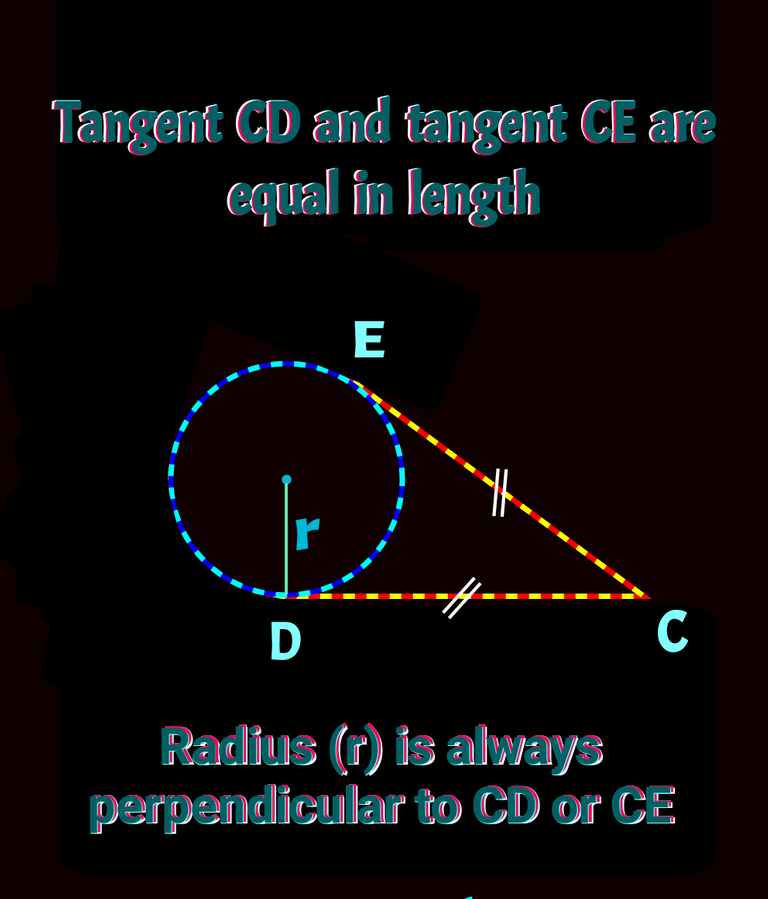
☑️☑️ The second thing we need here is the legendary Pythagoras theorem:
Hypotenuse² = Perpendicular² + Base². Details
LET'S PROVE ∆ = ar( a - r)/( a - 2r) :
As we know common tangents from outer point of a circle are equal in length, we can have three pairs of common tangents from three outer points (A, B & C) of O-centric in-circle with radius r. Check the following figure: 👇

Now, from right ∆ABC above, we can say
AB² + BC² = AC²
Or, (x + r)² + a² = {x + (a - r)}²
Or, x² + r² + 2rx + a² = x² + (a - r)² + 2x(a -r)
Or, r² + 2rx + a² = (a - r)² + 2ax - 2rx
[ x² is removed from both side ]
Or, r² + a² + 4rx = a² + r² -2ar +2ax
Or, 4rx = 2ax - 2ar
[(a² + r²) is removed from both side]
Or, 2rx = ax - ar [ Dividing both side by 2]
Or, 2rx - ax = - ar
Or, ax - 2rx = ar
Or, x(a - 2r) = ar
Or, x = ar/(a - 2r)
LET'S GO TO THE FIGURE AGAIN
Height AB = (x + r) unit = r + ar/(a - 2r) unit
Base BC = a
Ar. ∆ABC = 1/2 × BC × AB unit²
Or, ∆= 1/2 × a × { r + ar/(a - 2r)} unit ²
Check the following figure now :

🎤🎤All the figures and drawing are made by me using android only. There may be some silly mistakes; excuse me for that and the figure may not be accurate ; please try considering the data only.
Thank you so much guys for stoping by
I hope you have liked it
Have a great day
All is well
Regards: @meta007
Another good presentation.
!discovery 41
Thanks man for the appreciation.
LUV!
This post was shared and voted inside the discord by the curators team of discovery-it
Join our Community and follow our Curation Trail
Discovery-it is also a Witness, vote for us here
Delegate to us for passive income. Check our 80% fee-back Program
Thanks for your contribution to the STEMsocial community. Feel free to join us on discord to get to know the rest of us!
Please consider delegating to the @stemsocial account (85% of the curation rewards are returned).
Thanks for including @stemsocial as a beneficiary, which gives you stronger support.